Computational Methods in Reactor Physics
In nuclear reactors the neutron distribution is of primary importance for the power distribution and thermal analysis and fuel burnup. This distribution is intimately coupled with the thermal hydraulics of the systems due to inherent feedbacks. This gives rise to challenging problems in the analysis of reactor systems, especially under accident conditions where rapid excursions may take place. In our group we investigate such multiphysics phenomena by computational means. We also apply our techniques to other areas of science and engineering where radiation is an important factor (radiotherapy and electron microscopy).
Modal Analysis of Subcritical Systems (funded by EU)
Subcritical systems are nuclear reactors that cannot by themselves sustain a fission chain reaction and therefore require a neutron source to operate. Being subcritical, they have inherent advantages from a safety point of view and they are envisaged to be used for the ‘burning’ of nuclear waste. The dynamics of these systems under transient conditions is quite different from critical reactors. Also the subcriticality needs to be monitored to ensure safety during operation. With the help of numerical codes we try to understand the fundamental behavior of subcritical systems and also to optimize the measurement of the subcriticality level by searching for optimal detector locations.

The VENUS subcritical experimental reactor at SCK.CEN (Belgium)
Uncertainty Analysis of Multiphysics Systems (funded by EU and NRG)
The modeling of nuclear reactor systems is based on complex multiphysics models containing many input data such as detailed geometry and nuclear data. It is important to see the effect of uncertainties of such input data on important system characteristics such as maximum temperatures. The so-called best-estimate + uncertainties’ is also the new common approach in regulation. In our research we further develop the capabilities to efficiently perform sensitivity analysis and we also extensively utilize these methods in our research on Generation-IV reactors.
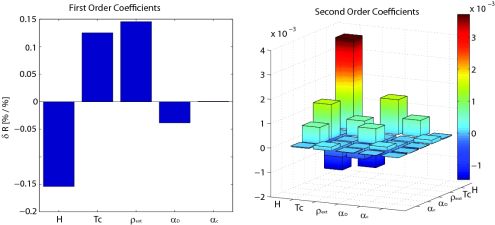
First and second-order sensitivity coefficients for the sensitivity of the reactor power obtained from an adjoint analysis procedure for a point kinetic model.
Adaptive Methods in Radiation Transport
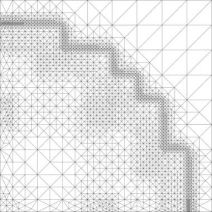

Computational mesh obtained after adaptive refinement (left) and savings obtained compared to a situation when no refinement is used.
Radiation transport can easily be the most computationally intensive part of a reactor simulation. In our work we try to make radiation transport tools more efficient by making use of adaptive refinement which focuses effort on regions that are most important in obtaining accurate answers. In such approaches the meshes (spatial, angular or energy) are selectively refined in regions that have more solution detail thereby saving computational effort in regions that are less demanding.
Charged Particle Transport for Proton Therapy
Treatment planning is currently based on optimization schemes that utilize approximate dose calculation algorithms. Such dose algorithms may behave inaccurate in heterogeneous tissue. Monte Carlo methods that are capable of accurate predictions are considered too expensive. Our research focuses on the development of deterministic solution methods for the radiation field for proton therapy and the resulting dose distribution in the patient. The methods we develop are able to deliver the accuracy of Monte Carlo but at less computational expense. It further allows for sensitivity analyses which are difficult with presently used dose engines.
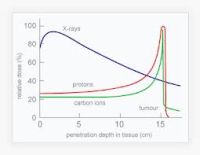
Dose distribution from a mono-energetic beam in human tissue. The Bragg peak can be used to localize deposited dose in the tumor region while sparing healthy tissue.